ASD of Concrete Masonry Lintels Based on the 2012 IBC/2011 MSJC

INTRODUCTION

Lintels and beams are horizontal structural members designed to carry loads above openings. Although lintels may be constructed of grouted and reinforced concrete masonry units, precast or cast-in-place concrete, or structural steel, this TEK addresses reinforced concrete masonry lintels only. Concrete masonry lintels have the advantages of easily maintaining the bond pattern, color, and surface texture of the surrounding masonry and being placed without need for special lifting equipment.

Concrete masonry lintels are sometimes constructed as a portion of a continuous bond beam. This construction provides several benefits: it is considered to be more advantageous in high seismic areas or areas where high winds may be expected to occur; control of wall movement due to shrinkage or temperature differentials is more easily accomplished; and lintel deflection may be substantially reduced.

The content presented in this TEK is based on the requirements of the 2012 IBC (ref. 1a), which in turn references the 2011 edition of the MSJC Code (ref. 2a).

Significant changes were made to the allowable stress design (ASD) method between the 2009 and 2012 editions of the IBC. These are described in detail in TEK 14-07C, ASD of Concrete Masonry (2012 IBC & 2011 MSJC) (ref. 3), along with a detailed presentation of all of the allowable stress design provisions of the 2012 IBC.

DESIGN LOADS

Vertical loads carried by lintels typically include:

1. distributed loads from the dead weight of the lintel, the dead weight of the masonry above, and any floor and roof loads, dead and live loads supported by the masonry; and
2. concentrated loads from floor beams, roof joists, or other beams framing into the wall. Axial load carried by lintels is negligible.

Most of these loads can be separated into the four types illustrated in Figure 1: uniform load acting over the effective span; triangular load with apex at mid-span acting over the effective span; concentrated load; and uniform load acting over a portion of the effective span.

The designer calculates the effects of each individual load and then combines them using superposition to determine the overall effect, typically by assuming the lintel is a simply supported beam.

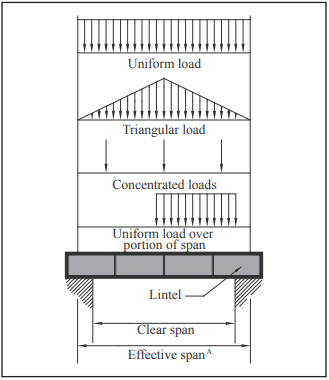
^A Effective span length is the center-to-center distance between supports.

Arching Action

For some configurations, the masonry will distribute applied loads in such a manner that they do not act on the lintel. This is called arching action of masonry. Arching action can be assumed when the following conditions are met (see also Figure 2):

• masonry wall laid in running bond,
• sufficient wall height above the lintel to permit formation of a symmetrical triangle with base angles of 45° from the horizontal as shown in Figure 2,
• at least 8 in. (203 mm) of wall height above the apex of the 45° triangle,
• minimum end bearing (4 in. (102 mm) typ.) is maintained,
• control joints are not located adjacent to the lintel, and
• sufficient masonry on each side of the opening to resist lateral thrust from the arching action.

Lintel Loading

The loads supported by a lintel depend on whether or not arching action can occur. When arching is not present, the lintel self-weight, the full weight of the wall section above the lintel and superimposed loads are considered. Self weight is a uniform load based on lintel weight (see Table 1).

When arching occurs, the wall weight supported by the lintel is taken as the wall weight within the triangular area below the apex (see Figure 2 and Table 2). This triangular load has a base equal to the effective span length of the lintel and a height of half the effective span. Any superimposed roof and floor live and dead loads outside this triangle are neglected, since they are assumed to be distributed to the masonry on either side of the lintel. Loads applied within the triangle need to be considered, however.

Concentrated loads are assumed to be distributed as illustrated in Figure 3. The load is then resolved onto the lintel as a uniform load, with a magnitude determined by dividing the concentrated load by this length. In most cases, this results in a uniform load acting over a portion of the lintel span.

The MSJC (ref. 2) does not address how to apply uniform loads that are applied within the 45° triangle. There are two schools of thought (see Figure 4):

1. Apply the full uniform load directly to the lintel without further distribution just as though there was no arching for those loads.
2. Distribute the portions of uniform loads that are applied within the 45o triangle to the lintel. These uniform loads within the 45o triangle may be dispersed and distributed at a 45o angle onto the lintel (ref. 5).

Lintels are required to be designed to have adequate stiffness to limit deflections that would adversely affect strength or serviceability. In addition, the deflection of lintels supporting unreinforced masonry is limited to the clear lintel span divided by 600 to limit damage to the supported masonry (ref. 2).

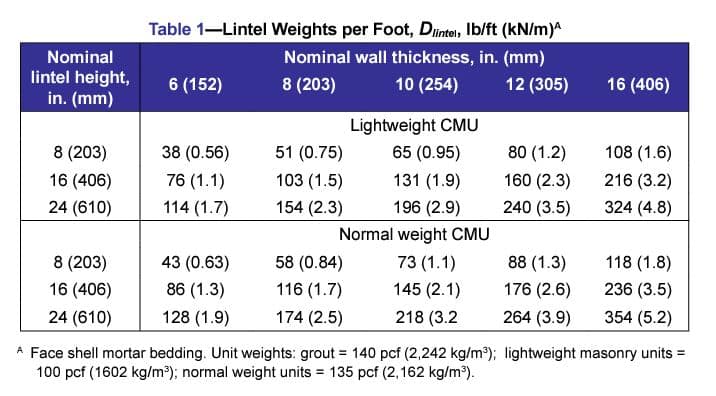
^A Face shell mortar bedding. Unit weights: grout = 140 pcf (2,242 kg/m³); lightweight masonry units = 100 pcf (1602 kg/m³); normal weight units = 135 pcf (2,162 kg/m³).

DESIGN TABLES

Tables 3 and 4 present allowable shear and moment, respectively, for various concrete masonry lintels, with various amounts of reinforcement and bottom cover based on a specified compressive strength of masonry, f’m = 1,500 psi (10.3 MPa) and the allowable stress design provisions of the 2011 MSJC (ref. 2a) and the 2012 IBC (ref.1a).

DESIGN EXAMPLE

Design a lintel for a 12 in. (305 mm) normal weight concrete masonry wall laid in running bond with vertical reinforcement at 48 in. (1.2 m) o.c. The wall configuration is shown in Figure 5.

Case 1—Arching Action

Check for Arching Action. Determine the height of masonry required for arching action. Assuming the lintel has at least 4 in. (102 mm) bearing on each end, the effective span is:

L = 5.33 + 0.33 = 5.67 ft (1.7 m).

The height of masonry above the lintel necessary for arching to occur in the wall (from Figure 2) is h + 8 in. (203 mm) = L/2 + 8 in. = 3.5 ft (1.1 m).
Based on an 8-in. (203-mm) high lintel, there is 18.0 – (3.33 + 4.0 + 0.67) = 10.0 ft (3.0 m) of masonry above the lintel. Therefore, arching is assumed and the superimposed uniform load is neglected.

Design Loads. Because arching occurs, only the lintel and wall dead weights are considered. Lintel weight, from Table 1, for 12 in. (305 mm) normal weight concrete masonry units assuming an 8 in. (203 mm) height is D_lintel = 88 lb/ft (1.3 kN/m).

For wall weight, only the triangular portion with a height of 3.5 ft (1.1 m) is considered. From Table 2, wall dead load is:

D_wall = 63 lb/ft² (3.5 ft)
= 221 lb/ft (3.2 kN/m) at the apex.

Maximum moment and shear are determined using simply supported beam relationships. The lintel dead weight is considered a uniform load, so the moment and shear are,

M_lintel = D_lintelL²/8
= (88)(5.7)²/8
= 357 lb-ft (0.48 kN-m)
V_lintel = D_lintelL/2
= (88)(5.7)/2 = 251 lb (1.1 kN)

For triangular wall load, moment and shear are,

M_wall = D_wallL²/12
= (221)(5.7)²/12
= 598 lb-ft (0.81 kN-m)
V_wall = D_wallL/4
= (221)(5.7)/4 = 315 lb (1.4 kN)

Because the maximum moments for the two loading conditions occur in the same locations on the lintel (as well as the maximum shears), the moments and shears are superimposed and summed:

M_max = 357 + 598
= 955 lb-ft = 11,460 lb-in (1.3 kN-m)
V_max = 251 + 315
= 566 lb (2.5 kN)

Lintel Design. From Tables 3 and 4, a 12 x 8 lintel with one No. 4 (M#13) bar and 3 in. (76 mm) or less bottom cover has adequate strength (M_all = 22,356 lb-in. (2.53 kN-m) and V_all = 2,152 lb (9.57 kN)). In this example, shear was conservatively computed at the end of the lintel. However, Building Code Requirements for Masonry Structures (ref. 2) allows maximum shear to be calculated using a distance d/2 from the face of the support.

Case 2—No Arching Action

Using the same example, recalculate assuming a 2 ft (0.6 m) height from the bottom of the lintel to the top of the wall. For ease of construction, the entire 2 ft (0.6 m) would be grouted solid, producing a 24 in. (610 mm) deep lintel.

Because the height of masonry above the lintel is less than 3.5 ft (1.1 m), arching cannot be assumed, and the superimposed load must be accounted for.

D_lintel = 264 lb/ft (3.9 kN/m), from Table 1. Because the lintel is 24 in. (610 mm) deep, there is no additional dead load due to masonry above the lintel.

W_total = 264 lb/ft + 1,000 lb/ft
= 1,264 lb/ft (18.4 kN/m)

M_max = wL²/8
= (1,264)(5.7)²/8 x 12 in./ft
= 61,601 lb-in (7.0 kN-m)

V_max = wL/2 = (1,264)(5.7)/2
= 3,602 lb (16.0 kN)

From Tables 3 and 4, a 12 x 24 lintel with one No. 4 (M#13) reinforcing bar and 3 in. (76 mm) or less bottom cover is adequate (M_all = 122,872 lb-in. (13.88 kN-m) and V_all = 10,256 lb (45.62 kN).

NOTATIONS

b           = width of lintel, in. (mm)
D_lintel   = lintel dead load, lb/ft (kN/m)
D_wall    = wall dead load, lb/ft (kN/m)
d           = distance from extreme compression fiber to centroid of tension reinforcement, in. (mm)
f’_m         = specified compressive strength of masonry, psi (MPa)
h            = half of the effective lintel span, L/2, ft (m)
L            = effective lintel span, ft (m)
M_all       = allowable moment, in.-lb (N⋅m)
M_lintel   = maximum moment due to lintel dead load, in.-lb (N⋅m)
M_max    = maximum moment, in.-lb (N⋅m)
M_wall    = maximum moment due to wall dead load moment, in.-lb (N⋅m)
V_all        = allowable shear, lb (N)
V_lintel    = maximum shear due to lintel dead load, lb (N)
V_max     = maximum shear, lb (N)
V_wall     = maximum shear due to wall dead load, lb (N)
W_total   = total uniform live and dead load, lb/ft (kN/m)
w          = uniformly distributed load, lb/in. (N/mm)

REFERENCES

1. International Building Code. International Code Council.
   1. 2012 Edition
2. Building Code Requirements for Masonry Structures. Reported by the Masonry Standards Joint Committee. a. 2011 Edition: TMS 402-11/ACI 530-11/ASCE 5-11
3. ASD of Concrete Masonry (2012 IBC & 2011 MSJC), TEK 14-07C, Concrete Masonry & Hardscapes Association, 2011.
4. Weights and Section Properties of Concrete Masonry Assemblies, CMU-TEC-002-23, Concrete Masonry & Hardscapes Association, 2023.
5. Openings in Concrete Masonry Walls (Part 1), Masonry Chronicles Winter 2008-09, Concrete Masonry Association of California and Nevada, 2009.